.
Team of more than 80 mathematicians from 12 countries has begun charting the terrain of rich, new mathematical worlds, and sharing their discoveries on the Web. The mathematical universe is filled with both familiar and exotic items, many of which are being made available for the first time. The "L-functions and Modular Forms Database," abbreviated LMFDB, is an intricate catalog of mathematical objects and the connections between them.
Making those relationships visible has been made possible largely by the coordinated efforts of a group of researchers developing new algorithms and performing calculations on an extensive network of computers. The project provides a new tool for several branches of mathematics, physics, and computer science.
A "periodic table" of mathematical objects
Project member John Voight, from Dartmouth College, observed that "our project is akin to the first periodic table of the elements. We have found enough of the building blocks that we can see the overall structure and begin to glimpse the underlying relationships." Similar to the elements in the periodic table, the fundamental objects in mathematics fall into categories.
Those categories have names like L-function, elliptic curve, and modular form. The L-functions play a special role, acting like 'DNA' which characterizes the other objects. More than 20 million objects have been catalogued, each with its L-function that serves as a link between related items. Just as the value of genome sequencing is greatly increased when many members of a population have been sequenced, the comprehensive material in the LMFDB will be an indispensible tool for new discoveries.
The LMFDB provides a sophisticated web interface that allows both experts and amateurs to easily navigate its contents. Each object has a "home page" and links to related objects, or "friends." Holly Swisher, a project member from Oregon State University, commented that the friends links are one of the most valuable aspects of the project: "The LMFDB is really the only place where these interconnections are given in such clear, explicit, and navigable terms. Before our project it was difficult to find more than a handful of examples, and now we have millions."
Experimental mathematics for the 21st century
Mathematics has always been an experimental science: conjectures are formulated and tested based on evidence of all kinds. As we enter a new century of research, large-scale computer experiments now take the place of hand calculations, with the effect of accelerating the process of testing and discovery.
John Jones, from Arizona State University, described this motivation for the project: "Many of us have made extensive computations, and we wanted to make this data available to other researchers and to link these projects together to aid mathematical progress. By joining forces, we now have a site for one-stop shopping of big data."
Many of these calculations are so intricate that only a handful of experts can do them, and some computations are so big and take so long that it makes sense to only do them once. The LMFDB also includes an integrated knowledge database that explains its contents and the mathematics behind it. Project member Brian Conrey, Director of the American Institute of Mathematics, thinks that the LMFDB approach is the wave of the future: "We are mapping the mathematics of the 21st century. The LMFDB is both an educational resource and a research tool which will become indispensable for future exploration."
According to Benedict Gross, an emeritus professor of mathematics at Harvard University, "Number theory is a subject that is as old as written history itself. Throughout its development, numerical computations have proved critical to discoveries, including the prime number theorem, and more recently, the conjecture of Birch and Swinnerton-Dyer on elliptic curves.
"During the past fifty years, modular forms and their L-functions have taken center stage at the forefront of number theory. The LMFDB pulls together all of the amazing computations that have been done with these objects. Having this material accessible in a single place will provide an invaluable resource for all of us working in the field."
Prime numbers have fascinated mathematicians throughout the ages. The distribution of primes is believed to be random, but proving this remains beyond the grasp of mathematicians to date. Under the Riemann hypothesis, the distribution of primes is intimately related to the Riemann zeta function, which is the simplest example of an L-function.
The LMFDB contains more than twenty million L-functions, each of which has an analogous Riemann hypothesis that is believed to govern the distribution of wide range of more exotic mathematical objects. Patterns found in the study of these L-functions also arise in complex quantum systems, and there is a conjectured to be direct connection to quantum physics.
Massive computations in the cloud
The scale of the computational effort involved in the LMFDB is staggering: a total of nearly a thousand years of computer time spent on calculations by multiple teams of researchers. A recent contribution by Andrew Sutherland at MIT used 72,000 cores of Google's Compute Engine to complete in one weekend a tabulation that would have taken more than a century on a single computer. As noted by Sutherland, "computations in number theory are often amenable to parallelization, and this makes it easy to scale them to the cloud." The application of large-scale cloud computing to research in pure mathematics is just one of the ways in which the project is pushing forward the frontier of mathematics.
History of the LMFDB
The idea of pooling the computational results of researchers in several areas of mathematics was started at a workshop at the American Institute of Mathematics in 2007. Work on the LMFDB began at a workshop supported by the National Science Foundation (NSF) in 2010.
The majority of the work on the LMFDB was done at subsequent workshops supported by the NSF and by the Engineering and Physical Sciences Research Council (EPSRC) in the UK through a Programme Grant awarded jointly to Warwick and Bristol Universities, as well as at long-term programs at the Mathematical Sciences Research Institute (MSRI) in Berkeley, CA, and the Institute for Computational and Experimental Research in Mathematics (ICERM) in Providence, RI. More than 100 research papers reference the data in the LMFDB.
.
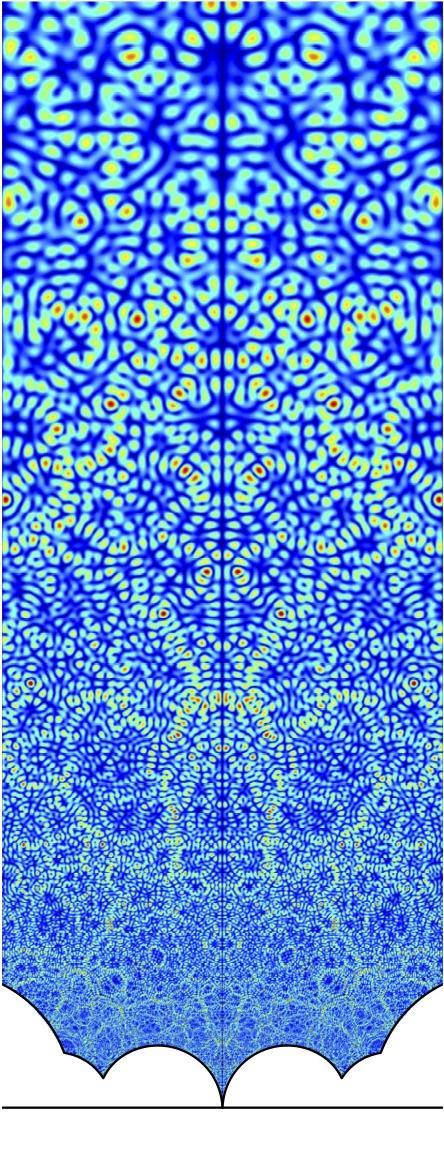
A nonholomorphic modular form is one of the many types of objects in the LMFDB. Image courtesy Fredrik Stroemberg.
Quelle: SD
4171 Views
